Reciprocating Engine Analysis by EXCEL

It was the highest priority to find out what is the power output of the reciprocating engine using gas generated from Wood Gasification Reactor. For this purpose, thermodinamic calculation was conducted for the gasoline engine installed on the  Wood Buggy.  Then power output for the gas fuel case was calculated applying the same (Actual Thermal Efficiency)/(Theoretical Efficiency) of the gasoline fuel case.

Internal combustion engine is an open cycle as shown in the following PV diagram.

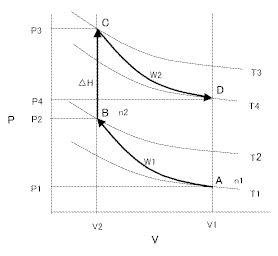
PV diagram

Ideal Gas was assumed. C_p were calculator from the same equations and parameter used in Gasification Reactor design. Arithmetic average temperature was used for C_p calculation. Direct Goal Seek method was used for convergence of the outlet temperature.

P (atm)

V (m³/kgmol)

n (kgmol/h)

R = 0.0887 (kcal/Nm³ deg.K)

T (deg K)

C_p - C_v = R

r = C_p / C_v = C_p / (C_p - R)

m = r (polytropic efficiency)    (m > 1)

Compression process (A to B) is described as follows. Gasoline was assumed to stay as liquid during compression process. Therefore, n1 is only intake air. In the gas fuel case, n1 is gas and air mixture. Joule-Thomson Effect at throttle valve was assumed zero because the gas was assumed as ideal gas. Polytropic efficiency of 100% was taken for calculating theoretical efficiency.

P₁V₁^m = P₂V₂^m

T₂/T₁ = (P₂/P₁)^{(m - 1)/m}

W₁= 24.2179 n₁(P₂V₂ - P₁V₁) / (m - 1)

Combustion process (B to C) is described as follows. It was assumed that all gasoline evaporate in this stage. DE_phase is the enthalpy change of this vaporization. DH is the heating value. n₂ is moral flow of exhaust gas.

DH - DE_phase = 24.2179 n₂ Cp (T₃ - T₂)

T₂ / P₂ = T₃ /P₃

Expansion process(C to D) is described as follows. Polytropic efficiency of 90% was taken.

P₃V₂^m = P₄V₁^m

T₄/T₃ = (P₄/P₃)^{(m - 1)/m}

W₂ = 24.2179 n₂ (P₃V₂ - P₄V₁) / (m - 1)

All in all,

Theoretical Thermal Efficiency = (W₂ - W₁)/ DH

(Actual Efficiency) / (Theoretical Efficiency)

It was assumed that (Actual Efficiency) / (Theoretical Efficiency) is specific for each machine and constant for different operational mode. It was also assumed that engine is driving electric generator and running at constant speed.

Calculation results for power generation purpose are shown below.

This program also can simulate Miller cycle engine by taking P₂/P₁=10 and P₃/P₄=13.

May 6, 2003

Rev. January 24, 2010